Technical Notes
Small Gadgets in Research
- VBA code to insert brackets around text in Excel (code)
- Subplot function to plot multiple plots in Matplotlib with Python (code and sample data) (text)
Useful Tools and Materials (External Links)
- A Gentle Introduction to Graph Neural Networks (Google Research)
- A Preprocessor for Function Definitions in GAMS (Ferris, Rutherford and Starkweather)
- An Introduction to QGIS and A Walkthrough of How to Create the Athens Map (Liang)
- A Theory of First-Order Counterfactual Reasoning (Burgard, Christaller and Cremers)
- Calibration and Counterfactuals of Gravity Trade Models (Allen and Arkolakis)
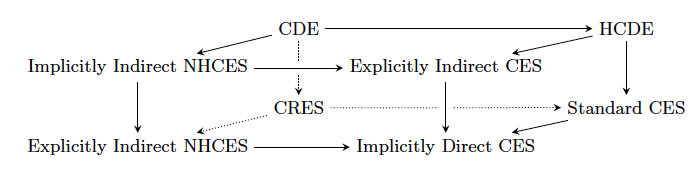
- CES Functions: Some Hints and Useful Formulae (Rutherford)
- Counterfactual Theories of Causation (Stanford Encyclopedia of Philosophy)
- Demand Estimation and Industrial Organization Papers Collection (Matthew Shum)
- Discrete Choice Methods with Simulation (Kenneth Train)
- Estibration: An Illustration of Structural Estimation As Calibration (Balistreri and Hillberry)
- Exact Hat Algebra used in Deckle, Eaton and Kortum (Dingel)
- Exact Hat and MCP solution with the CES Demand - a Comparison (Yang)
- Examples of using Replaceable Parameters in CMF files (Horridge and Jerie)
- Mixed Integer Nonlinear Program: Model with Both Nonlinearity and Discrete Variables (Vigerske)
- Optimal Transport: Theory and Application (James M. Murphy)
- Performing Multiple Simulations using CMF Parameters with GEMPACK 11.2: the Mystery of Horridge's Kink (Jerie)
- The Economics of Space (Arkolakis)